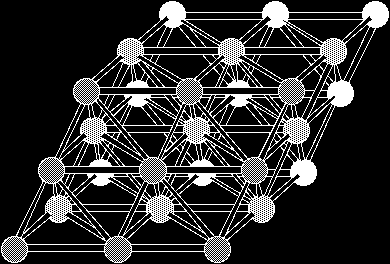
An important aspect of approaches to solving problems is the representation scheme employed for knowledge about the problem. We propose a particular vivid, analogical representation for solving problems in the spatial/kinematic domain: lattice computer architectures and associated algorithms.

We argue, partly with evidence from the Clinical and Experimental Psychology and Artificial Intelligence literatures, that our approach is superior to approaches using sentential representations

The physical sciences provide problems in the spatial/kinematic domain as do many puzzles and situations ostensibly requiring common sense. Solutions to such problems, at least from the physical sciences, are usually predictions of events in the course of the unfolding of a phenomenon under consideration, e.g., predictions of the motions of the participating objects. For spatial puzzle solving, the finding of solutions might involve some trial and error motion of objects in space rather than the tracking of deterministic simulations of physical phenomena. In any case, the knowledge-base for solving spatial problems is the representation of the objects participating, their motions, and the, usually finite, region of space in which the ``action'' takes place or is to take place. The focus of this ongoing project is to investigate discrete structures for representing (finite regions) of euclidean space and design algorithms for the analog simulation of physical phenomena in this discrete universe. Click on ``The Methodology'' below to see a pictorial description of our methodology.

Most physical phenomena involve the spatial/kinematic domain. Hence, our approach may be well-suited for scientific computing Scientific computing provides but a subset of the problems we have in mind for our approach.

This page maintained by Anil M. Shende (shende@abdabs.roanoke.edu)